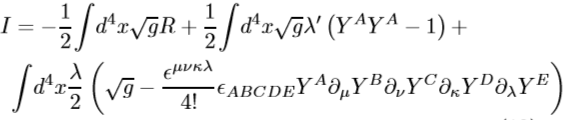Search This Blog
Pilgrimage of a physicist, peri-mathematician, & proto-philosopher looking for a trace of reality in a maze of quantum speculations staying tuned in to the spectral information provided by experimental physics and noncommutative geometry.
Posts
Showing posts from September, 2014
Epistemology and phenomenology of B-L gauged extension of the Standard Model
- Get link
- Other Apps
Our (quantum and gravitating) physical universe from a conservative (neither compulsory stringy nor mandatory supersymmetric) viewpoint
- Get link
- Other Apps
Is the classically conformal B-L extended Standard Model a minimal spectral standard model in disguise?
- Get link
- Other Apps
How to save (or rather softly break) supersymmetry with the help of noncommutative geometry?
- Get link
- Other Apps
Parallel but opposite or dual and complementary histories of two different ideas?
- Get link
- Other Apps
There are more things in theory and phenomenology, my friend, than are covered in more popular blogs.
- Get link
- Other Apps
Successive slidings of SUSY looking for a better place...
- Get link
- Other Apps
A spectral non commutative shot in the darkness of quantum physics and Einstein's gravity
- Get link
- Other Apps
Heisenberg relation(s) for spectral non commutative spacetime?
- Get link
- Other Apps
1814-2014 : 200 years of spectroscopy / 200 ans de spectroscopie
- Get link
- Other Apps